There’s a rule-of-thumb that for every equation that you use in writing you lose 90% of your audience.  So to the less than one percent of you that remain after my last blog, I’d like to point out that by determining the magnification of a camera lens, we are finally in a position to begin to address the very import questions: how sharp and image can we hope to achieve?

We showed in the previous post that the magnification, or actually demagnification of a lens is approximately the focal length divided by the distance from the camera to what you are photographing, the so called object distance.  This is great.

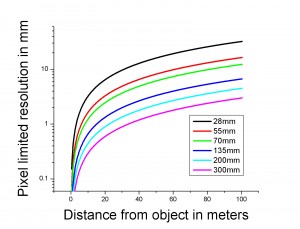
Pixel limited resolution, the smallest thing that you could see, for different lenses as a function of distance from the object

Now recognize that if you had some kind of perfect lens your resolution would be limited by the pixel spacing in your detector.  Remember that the pixels are like a graph paper on which your image is projected.  We can ask a very simple question.  How far apart need two white spots be to be distinguished in a photograph.  Well, there has to be a back spot in between otherwise they would merge into one.  For my Canon T2i this is 8.6 um (that’s .0086 mm). So an estimate of image resolution is to calculate the magnification as a function of distance and then divide the .0086 mm by the magnification to determine the equivalent separation in at the object.  I’m calling this number the pixel limited resolution.

I’ve done this for you in the figure, for a set of typical lens focal lengths.  It’s pretty impressive. Even at 100 m, more than the length of a football field, you would be able to resolve 3 mm.  Effectively we are looking at the finest detail that you could see if your camera’s resolution were pixel limited.  Is it pixel limited?  Well stay tuned!